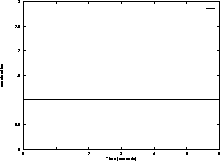
Figure 2.2: Acceleration of a car vs. Time
Calculus arose out of a need to describe the motion of everyday objects. To use a modern example, consider an automobile. The driver holds the accelerator pedal down at a steady level, as represented by the graph of figure 2.2:

Figure 2.2: Acceleration of a car vs. Time
With the accelerator depressed at a constant level, the speed of the vehicle should steadily increase (figure 2.3).
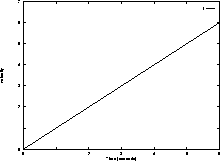
Figure 2.3: Velocity of the same car vs. Time
However, with a linearly increasing velocity as above, calculus tells us that our position relative to our starting point increases exponentially (figure 2.4).
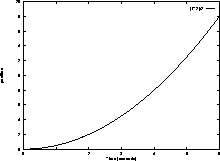
Figure 2.4: Position of the same car vs. Time
From the three concepts of displacement, velocity and acceleration came Calculus. It worked its magic by slicing time into infinitesimal slivers which are considered in efforts to deduce a greater understanding about the whole. A differential equation is a time sliver equation, a way of describing how a system changes over an infinitesimally small amount of time.
In 1713, Brook Taylor was able to solve a differential equation that described the movements of a violin string, to find that it had the familiar shape of a sine curve (Figure 2.5):
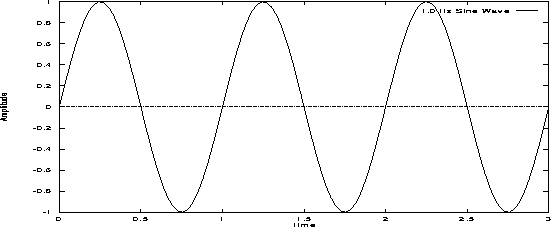
Figure 2.5: A 1.0 Hz Sine Wave
From that sine curve, whole branches of physics and mathematics were set in stone. Leonhard Euler, Daniel Bernoulli and Joseph Fourier began examining the waveforms that could be created from multiple sine waves being added together. Euler analyzed the sounds of bells and drums, Bernoulli worked on organ pipes and Fourier developed ``Fourier Analysis'' by which a great deal of musical signals can be described.
As the mathematicians began deterministically chipping away at the laws of the universe, each held firm in the belief that in this manner, all of the fundamental truths would soon be completely describable by mathematics, just the way it should be.
If only Mother Nature had intended it to be so easy.